Two circles with centers at with radii
for
are mutually tangent if
|
(1)
|
If the center of the second circle is inside the first, then the and
signs both correspond to internally tangent circles. If the
center of the second circle is outside the first, then the
sign corresponds to externally tangent circles and the
sign to internally tangent circles.
Finding the circles tangent to three given circles is known as Apollonius' problem. The Desborough Mirror, a beautiful bronze mirror made during the Iron Age between 50 BC and 50 AD, consists of arcs of circles that are exactly tangent (Wolfram 2002, pp. 43 and 873).
Given three distinct noncollinear points ,
,
and
,
denote the side lengths of the triangle
as
,
, and
. Now let three circles be drawn,
one centered about each point and each one tangent to the other two (left figure),
and call the radii
,
,
.
Interestingly, the pairwise external similitude centers of these circles are the three Nobbs points (P. Moses, pers. comm., Mar. 14, 2005).
These three circles satisfy
|
(2)
| |||
|
(3)
| |||
|
(4)
|
(right figure). Solving for the radii gives
|
(5)
| |||
|
(6)
| |||
|
(7)
|
Plugging these equations in to the equation of the semiperimeter of
|
(8)
|
gives
|
(9)
|
so
|
(10)
|
In addition,
|
(11)
|
Switching
and
to opposite sides of the equation and noting that the above argument applies equally
well to
and
then gives the radii of the three circles as
|
(12)
| |||
|
(13)
| |||
|
(14)
|
The pairwise points of tangency of the three circles are precisely the vertices of the contact triangle of
, i.e., the triangle formed by the points at which the
incircle is tangent to the original triangle. The circles that are internally and
externally tangent to these three circles are known as the Soddy
circles.
No Kimberling centers lie on any of the tangent circles.
The radical circle of the tangent circles is the incircle.
Two circles of radii
and
with centers separated by a distance
are externally tangent if
|
(15)
|
and internally tangent if
|
(16)
|
The following table summarizes tangent circles for some common named circles. As can be seen, the incircle, nine-point circle, and Moses circle are mutually tangent at the Feuerbach point.
| circle | tangent circles |
| incircle | nine-point circle |
| Moses circle | nine-point circle |
| nine-point circle | incircle, Moses circle |
There are four circles that are tangent all three sides (or their extensions) of a given triangle: the incircle and three excircles
,
, and
. These four circles are, in turn, all touched by the nine-point circle
.
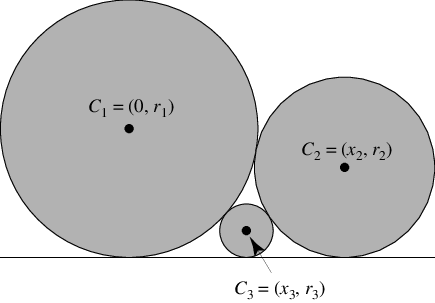
If two circles
and
of radii
and
are mutually tangent to each other and a line, then their centers are separated by
a horizontal distance given by solving
|
(17)
|
for ,
giving
|
(18)
|
The position and radius of a third circle tangent to the first two and the line can be found by solving the simultaneous equations
|
(19)
| |||
|
(20)
|
for
and
,
giving
 |
(21)
| ||
|
(22)
|
The latter equation can be written in the form
|
(23)
|
This problem was given as a Japanese temple problem on a tablet from 1824 in the Gumma Prefecture (Rothman 1998).