A deck transformation, also called a covering transformation, of a covering map 
is a homeomorphism 
satisfying 
.
For a connected universal cover 
,
choose a base point 
and a lift 
. Every loop 
based at 
has a unique lift ![f^~:[0,1]->X^~](/images/equations/DeckTransformation/Inline9.svg)
starting at 
. Its endpoint depends only on the homotopy
class of 
.
There is a unique deck transformation taking 
to this endpoint. This construction identifies the deck
transformation group 
with the based fundamental
group of 
.
Its group action on an arbitrary point 
is defined by this homeomorphism.
The loop-lifting construction applies directly only to points over 
.
The deck transformations of the universal cover act with quotient space
 |
For a graph cover 
, deck transformations are graph
automorphisms of 
that preserve the graph
projection 
.
A graph cover is a regular
graph cover if its deck transformations act transitively
on each fiber of 
.
For example, when 
is the square torus then 
is the plane and the preimage 
is a translation
of the integer lattice 
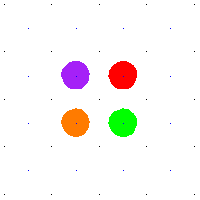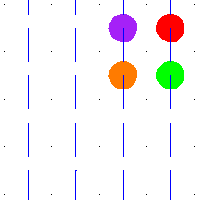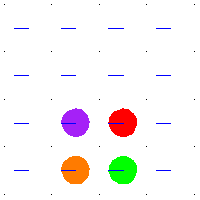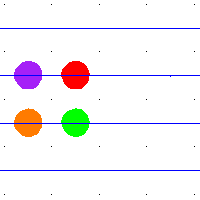
. Any loop based
at 
in the torus lifts to a path
in the plane, with the endpoints lying in the same translated
integer lattice. These translated integer lattices are the group
orbits of the group action of 
on 
by addition. The above animation
shows the group action of some deck transformations
on some disks in the plane. The
spaces are the torus and its
universal cover, the plane.
An element of the fundamental
group, shown as the path in blue, defines a deck transformation
of the universal cover. It moves around the points in the universal cover.
The points moved to have the same projection in the torus. The blue path is a loop
in the torus, and all of its preimages
are shown.
