Spikey

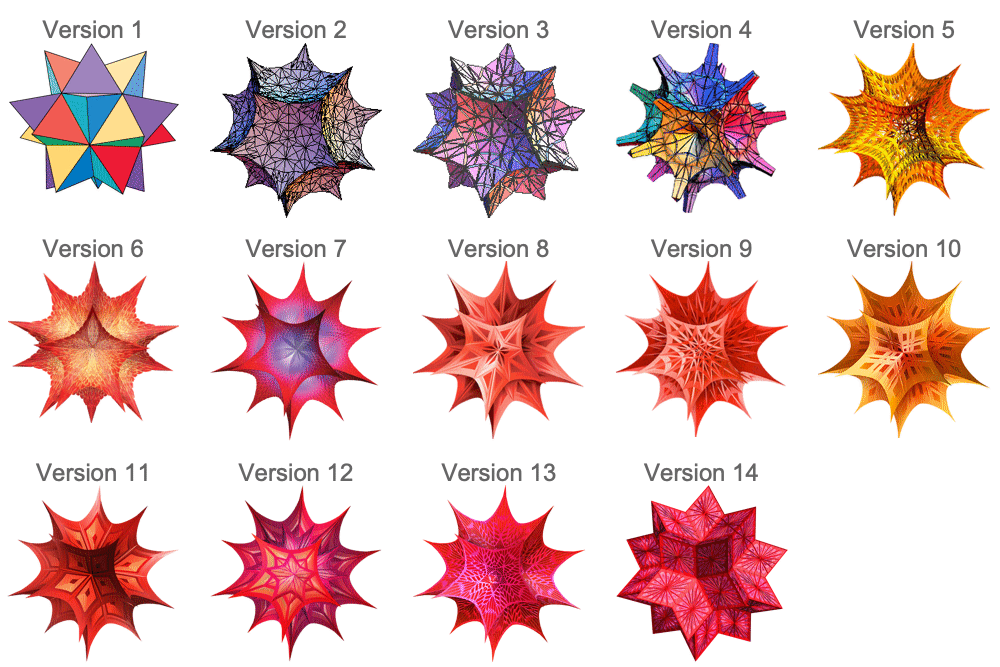
"Spikey" is the logo of Wolfram Research, makers of Mathematica and the Wolfram Language. In its original (Version 1) form, it is an augmented icosahedron with an augmentation height of , not to be confused with the great stellated dodecahedron, which is a distinct solid. This gives it 60 equilateral triangular faces, making it a deltahedron. More elaborate forms of Spikey have been used for subsequent versions of Mathematica. In particular, Spikeys for Version 2 and up are based on a hyperbolic dodecahedron with embellishments rather than an augmented icosahedron (Trott 2007, Weisstein 2009).

The "classic" (Version 1) Spikey illustrated above is implemented in the Wolfram Language as PolyhedronData["MathematicaPolyhedron"].

The skeleton of the classic Spikey is the graph of the triakis icosahedron.

A glyph corresponding to the classic Spikey, illustrated above, is available as the character \[MathematicaIcon] in the Wolfram Language.

The above image shows an origami Spikey consisting of 30 double-pocket equilateral modules (Fusè 1990, pp. 126-128).

The cross-stitched Spikey shown above was created by Jennifer Peterson (pers. comm., Dec. 5, 2006).

In August 2004, R. Bell has constructed a 300-400-pound gigantic Spikey sculpture out of plywood, illustrated above (photos courtesy of Merritt Pelkey). Bell's plywood Spikey has an internal pentagonal floor, external metal strapping to hold the equilateral triangles together into the constituent tetrahedra, and internal metal strapping to hold the tetrahedra together into the final structure. Metal struts line the bottom five edges to strengthen the base and prevent the bottom tips from crushing. Assembly requires about 6 hours.

The volume of the Spikey constructed from an icosahedron with unit edge lengths is

(1)

and the surface area is

(2)

The inertia tensor is given by the diagonal matrix with diagonal elements

(3)