A compact manifold is a manifold that is compact as a topological space. Examples are the circle (the only connected
one-dimensional compact manifold
without boundary) and the 
-dimensional sphere
and torus. Connected
compact manifolds in two dimensions
are completely classified by their orientation
and the number of holes (genus).
It should be noted that the term "compact manifold"
often implies "manifold without boundary,"
which is the sense in which it is used here. When there is need for a separate term,
a compact boundaryless manifold is called a closed
manifold.
For many problems in topology and geometry, it is convenient to study compact manifolds because of their "nice" behavior. Among the properties making compact manifolds "nice" are the fact that they can be covered by finitely many coordinate charts, and that any continuous real-valued function is bounded on a compact manifold.
For any positive integer 
, a distinct nonorientable
surface can be produced by replacing 
disks in the sphere
with Möbius strips. In particular, replacing
one disk with a Möbius
strip produces a cross surface and replacing
two disks produces the Klein
bottle. The sphere, the 
-holed tori, and this sequence
of nonorientable surfaces form a complete
list of connected compact, boundaryless two-dimensional manifolds. General
compact manifolds without boundary are finite disjoint unions of connected
compact manifolds.
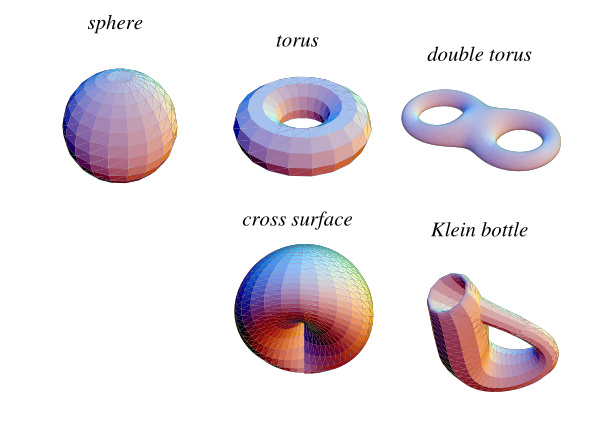
The following table lists the classes of connected two-dimensional compact manifolds
for small 
,
which are also illustrated above.
