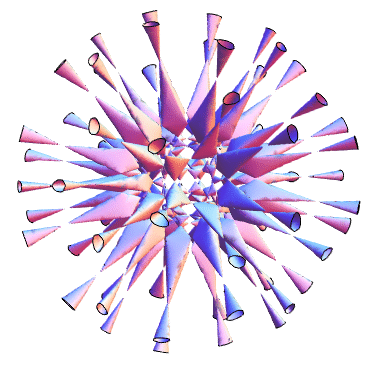
The Barth decic is a decic surface in complex three-dimensional projective space having the maximum possible number of ordinary double points, namely 345. It is given by the implicit equation
![8(x^2-phi^4y^2)(y^2-phi^4z^2)(z^2-phi^4x^2)(x^4+y^4+z^4-2x^2y^2-2x^2z^2-2y^2z^2)+(3+5phi)(x^2+y^2+z^2-w^2)^2[x^2+y^2+z^2-(2-phi)w^2]^2w^2
=0,](/images/equations/BarthDecic/NumberedEquation1.svg) |
where is the golden
ratio and
is a parameter.
The case ,
illustrated in the above plot, has 300 ordinary
double points. These 300 points lie symmetrically at the vertices of two 60-faced
and two 90-faced solids, illustrated above, with the 60-faces solids corresponding
to a truncation of the icosahedron (but not the symmetrically
truncated truncated icosahedron).
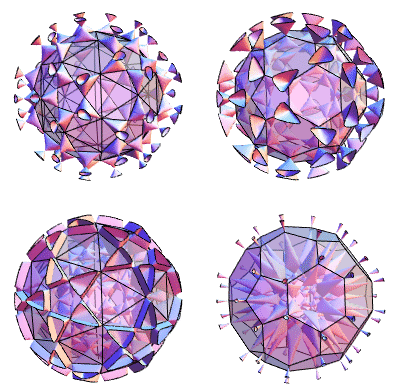
The concentric groups of points lie at distances ,
,
1, and
from the origin, illustrated above.
The Barth decic is invariant under the icosahedral group.