Analytic continuation (sometimes called simply "continuation") provides a way of extending the domain over which a complex
function is defined. The most common application is to a complex analytic
function determined near a point by a power series
 |
(1)
|
Such a power series expansion is in general valid only within its radius of convergence. However, under fortunate circumstances (that are very fortunately
also rather common!), the function will have a power series expansion
that is valid within a larger-than-expected radius
of convergence, and this power series can be
used to define the function outside its original domain
of definition. This allows, for example, the natural extension of the definition
trigonometric, exponential, logarithmic, power, and hyperbolic functions from the
real line
to the entire complex plane
. Similarly, analytic continuation can
be used to extend the values of an analytic function across a branch
cut in the complex plane.
Let
and
be analytic functions on domains
and
, respectively, and suppose that the intersection
is not empty
and that
on
.
Then
is called an analytic continuation of
to
, and vice versa (Flanigan 1983, p. 234). Moreover,
if it exists, the analytic continuation of
to
is unique.
This uniqueness of analytic continuation is a rather amazing and extremely powerful statement. It says in effect that knowing the value of a complex function in some finite complex domain uniquely determines the value of the function at every other point.
By means of analytic continuation, starting from a representation of a function by any one power series, any number of other power series can be found which together define the value of the function at all points of the domain. Furthermore, any point can be reached from a point without passing through a singularity of the function, and the aggregate of all the power series thus obtained constitutes the analytic expression of the function (Whittaker and Watson 1990, p. 97).
Analytic continuation can lead to some interesting phenomenon such as multivalued functions. For example, consider analytic continuation of the square
root function . Although this function is not globally well-defined
(since every nonzero number has two square roots),
has a well-defined Taylor series
around
,
|
(2)
| |||
|
(3)
|
which can be used to extend the domain over which is defined. Note that when
, the power series for
has a radius of convergence of 1.
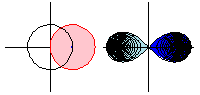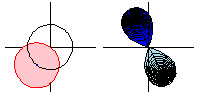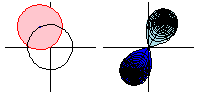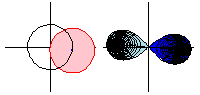
The animation above shows the analytic continuation of along the path
. Note that when the function goes all the way around,
is the negative of the original function, so going around twice returns the function
to its original value.
In the animation, the domain space (colored pink; left figures) is mapped to the image space (colored blue; right figures) by the square root function, and the light blue region indicates the negative square root. However, by continuing the function around the circle, the square root function takes values in what used to be the light blue region, so the roles of the blue and light blue region are reversed.
This can be interpreted as going from one branch of the multivalued square root function to the other. This illustrates that analytic continuation extends a function using the nearby values that provide the information on the power series.
It is possible for the function to never return to the same value. For example, increases by
every time it is continued around zero. The natural
domain of a function is the maximal chain of domains on which a function can
be analytically continued to a single-valued
function. For
, it is the connected infinite cover
of the punctured plane, and for
it is the connected double cover.
If there is a boundary across which the function cannot be extended, then is called
the natural boundary. For instance, there exists
a meromorphic function
in the unit disk where every point on the unit circle is a
limit point of the set of poles.
Then the circle is a natural boundary for
.