Fundamental Group

The fundamental group of a pathwise-connected topological space at a basepoint is the group of equivalence classes of loops based at , under homotopies that keep fixed. The identity element of this group is the equivalence class of the constant loop at . The fundamental groups of homeomorphic spaces are isomorphic. In fact, the fundamental group only depends on the homotopy type of . The fundamental group of a topological space was introduced by Poincaré (Munkres 1993, p. 1).

The following is a table of the fundamental group for some common spaces , where denotes the fundamental group, is the first integral homology group, denotes the group direct product, denotes the free product, denotes the additive group of integers, and is the cyclic group of order .

space ()	symbol
circle
complex projective space		0	0
figure eight
Klein bottle
-torus
real projective plane
sphere		0	0

If based loops and represent two group elements, their group product is represented by the loop followed by the loop . The identity element is represented by the constant path, and the inverse element of the class represented by is given by traversing in the opposite direction. For a pathwise-connected space, a chosen path between basepoints and induces an isomorphism between their fundamental groups. This isomorphism can depend on the chosen path, so the fundamental group of is independent of the basepoint up to isomorphism.

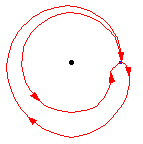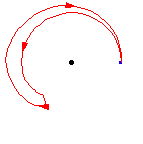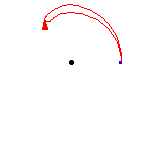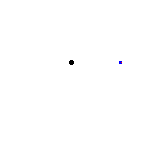
The diagram above shows that a loop followed by the opposite loop is homotopic to the constant loop, i.e., the identity element. That is, it starts by traversing the path , and then turns around and goes the other way, . The composition is deformed, or homotoped, to the constant path, along the original path .

A pathwise-connected space with a trivial fundamental group (i.e., every loop is homotopic to the constant loop), is called simply connected. For instance, any contractible space, like Euclidean space, is simply connected. The sphere is simply connected, but not contractible. When admits a universal cover , the covering space is simply connected, and loops in lift to paths in . The lifted paths in the universal cover define the deck transformations, which form a group isomorphic to the fundamental group.

The underlying set of the fundamental group of is the set of based homotopy classes from the circle to , denoted . For general spaces and , there is no natural group structure on . The spheres for , including the circle, are examples of co-H-spaces. The based homotopy classes from to form the homotopy groups . In general, the fundamental group is non-Abelian. However, the higher homotopy groups are Abelian. In some special cases, the fundamental group is Abelian. 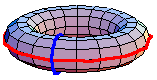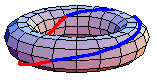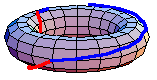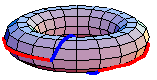For example, the animation above shows that in the torus. The red path goes before the blue path. The animation is a homotopy between the loop that goes around the inside first and the loop that goes around the outside first.

Taking the singular homology class of a based loop defines a group homomorphism

which is surjective. In fact, the group kernel of is the commutator subgroup and is called Abelianization.

The fundamental group of can be computed using van Kampen's theorem for an open cover by pathwise-connected spaces containing a common basepoint, with pathwise-connected pairwise and triple intersections, when the fundamental groups of the cover spaces and their pairwise intersections and the inclusion-induced homomorphisms are known.

A continuous map induces a group homomorphism by composing each based loop with . This construction is natural, i.e., whenever the function composition of the two maps is defined.