In general, a tetrakis hexahedron is a non-regular icositetrahedron that can be constructed as a positive augmentation of a cube. Such a solid is also known as a tetrahexahedron, especially to mineralogists (Correns 1949, p. 41; Berry and Mason 1959, p. 127). While the resulting icositetrahedron is not regular, its faces are all identical.
"The" tetrakis hexahedron is the 24-faced dual polyhedron of the truncated octahedron (Holden 1971, p. 55). It can be constructed by augmentation of a unit cube by a pyramid with height 1/4. It is illustrated above together with a wireframe version and a net that can be used for its construction.
It is Wenninger dual .
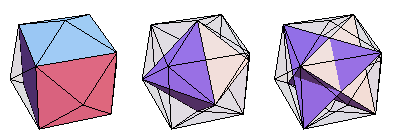
A cube, octahedron, and stella octangula can all be inscribed in the vertices of the tetrakis hexahedron (E. Weisstein, Dec. 25, 2009).
The edge lengths for the tetrakis hexahedron constructed as the dual of the truncated octahedron with unit edge lengths are
|
(1)
| |||
|
(2)
|
Normalizing so that
gives a tetrakis hexahedron with surface area and
volume
|
(3)
| |||
|
(4)
|
