The Steinmetz solid is the solid common to two (or three) right circular cylinders of equal radii intersecting at right angles is called the Steinmetz solid. Two cylinders intersecting at right angles are called a bicylinder or mouhefanggai (Chinese for "two square umbrellas"), and three intersecting cylinders a tricylinder. Half of a bicylinder is called a vault.
Closed forms exist for the volume of a cylinder-cylinder intersection where the cylinders are of different radii and intersect centrally
at arbitrary angle 
(Hubbell 1965).
 |  |
For two cylinders of radius 
oriented long the 
- and 
-axes gives the equations
 |
(1)
|
 |
(2)
|
which can be solved for 
and 
gives the parametric equations of the edges
of the solid,
 |  |  |
(3)
|
 |  |  |
(4)
|
The surface area can be found as 
, where
 |  |  |
(5)
|
 |  |  |
(6)
|
Taking the range of integration as a quarter or one face and then multiplying by 16 gives
 |
(7)
|
The volume common to two cylinders was known to Archimedes (Heath 1953, Gardner 1962) and the Chinese mathematician Tsu Ch'ung-Chih (Kiang 1972),
and does not require calculus to derive. Using calculus
provides a simple derivation, however. Noting that the solid has a square cross
section of side-half-length 
, the volume is given by
 |
(8)
|
(Moore 1974). The volume can also be found using cylindrical algebraic decomposition, which reduces the inequalities
 |
(9)
|
to
 |
(10)
|
giving the integral
 |
(11)
|
If the two right cylinders are of different radii 
and 
with 
, then the volume common to
them is
![V_2(a,b)=8/3a[(a^2+b^2)E(k)-(a^2-b^2)K(k)],](/images/equations/SteinmetzSolid/NumberedEquation8.svg) |
(12)
|
where 
is the complete elliptic integral
of the first kind, 
is the complete elliptic integral
of the second kind, and 
is the elliptic modulus.
Each of the curves of intersection of two cylinders of radii 
and 
is sometimes known as a Steinmetz
curve.
The volume common to two elliptic cylinders
 |
(13)
|
with 
is
![V_2(a,c;b,c^')=(8ab)/(3c)[(c^('2)+c^2)E(k)-(c^('2)-c^2)K(k)],](/images/equations/SteinmetzSolid/NumberedEquation10.svg) |
(14)
|
where 
(Bowman 1961, p. 34).
 |  |  |
For three cylinders of radii 
intersecting
at right angles, The resulting solid has 12 curved
faces. If tangent planes are drawn where the faces meet, the result is a rhombic
dodecahedron (Wells 1991). The volume of intersection
can be computed in a number of different ways,
 |  |  |
(15)
|
 |  |  |
(16)
|
 |  |  |
(17)
|
(Moore 1974). According to the protagonist Christopher in the novel The Curious Incident of the Dog in the Night-Time, "...People go on holidays to see new things and relax, but it wouldn't make me relaxed and you can see new things by looking at earth under a microscope or drawing the shape of the solid made when 3 circular rods of equal thickness intersect at right angles" (Haddon 2003, p. 178), which is of course precisely the Steinmetz solid formed by three symmetrically placed cylinders.
Four cylinders can also be placed with axes along the lines joining the vertices of a tetrahedron with the centers on the opposite sides. The resulting solid of intersection has volume
 |
(18)
|
and 24 curved faces analogous to a cube-octahedron compound (Moore 1974, Wells 1991).
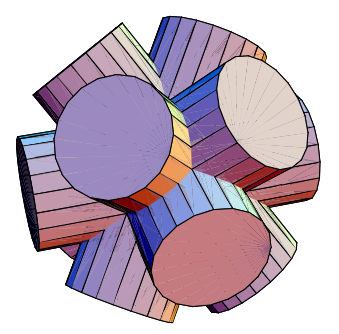
Six cylinders can be placed with axes parallel to the face diagonals of a cube. The resulting solid of intersection has volume
 |
(19)
|
and 36 curved faces, 24 of which are kite-shaped and 12 of which are rhombic (Moore 1974).
