|

|
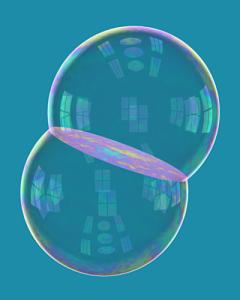
A double bubble is pair of bubbles which intersect and are separated by a membrane bounded by the intersection. The usual double bubble is illustrated in the left figure above. A more exotic configuration in which one bubble is torus-shaped and the other is shaped like a dumbbell is illustrated at right (illustrations courtesy of J. M. Sullivan).
In the plane, the analog of the double bubble consists of three circular arcs meeting in two points. It has been proved that the configuration of arcs meeting at equal
angles)
has the minimum perimeter for enclosing two equal areas
(Alfaro et al. 1993, Morgan 1995).
It had been conjectured that two equal partial spheres sharing a boundary of a flat disk separate two volumes of air using a total surface
area that is less than any other boundary. This equal-volume case was proved
by Hass et al. (1995), who reduced the problem to a set of integrals which they carried out on an ordinary PC. Frank
Morgan, Michael Hutchings, Manuel Ritoré, and Antonio Ros finally proved the
conjecture for arbitrary double bubbles in early 2000. In this case of two unequal
partial spheres, Morgan et al. showed that the separating boundary which minimizes
total surface area is a portion of a sphere which meets
the outer spherical surfaces at dihedral angles
of
. Furthermore, the curvature
of the partition is simply the difference of the curvatures
of the two bubbles,
|
(1)
|
where
is the radius of the interface and
and
are the radii of the bubbles (Isenberg 1992, pp. 88-95).
Furthermore, for three bubbles with radii
,
,
and
, and interface radii
, and
,
|
(2)
|
|
(3)
|
(Isenberg 1992, pp. 88-95).
Amazingly, a group of undergraduates has extended the theorem to four-dimensional double bubbles, as well as certain cases in five-space and higher dimensions. The corresponding triple bubble conjecture remains open (Cipra 2000).