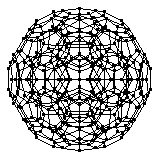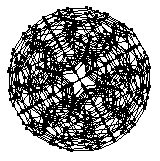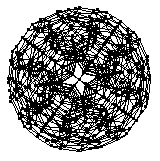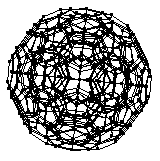
The 120-cell is a finite regular four-dimensional polytope with Schläfli symbol 
. It is also known as the hyperdodecahedron or hecatonicosachoron,
and is composed of 120 dodecahedra, with 3 to an
edge, and 720 pentagons (Coxeter 1973, p. 264).
The 120-cell has 600 vertices (Coxeter 1969) and 1200 edges. It is one of the six
regular polychora.
In the plate following p. 176, Coxeter (1973) illustrates the polytope.
The dual of the 120-cell is the 600-cell.
The vertices of the 120-cell with circumradius 
and edge length 
are given by the following sets, where 
is the golden ratio (Coxeter
1969, p. 404).
1. A set of 24 vectors given by 
and all its permutations.
2. A set of 64 given by 
and all its permutations.
3. A set of 64 given by 
and all its permutations.
4. A set of 64 given by 
and all its permutations.
5. A set of 96 given by 
and all its even permutations.
6. A set of 96 given by 
and all its even permutations.
7. A set of 192 given by 
and all its even permutations.
There are 30 distinct nonzero distances between vertices of the 120-cell in 4-space.
The top image above shows a projection of the 120-cell laser-etched into glass, and the two bottom images show a projection visualized as a metal sculpture. Both works were created by digital sculptor Bathsheba Grossman (https://www.bathsheba.com/).
The 120-cell graph is the skeleton of the 120-cell.
The 120-cell has
 |
distinct nets (Buekenhout and Parker 1998).
." Disc. Math. 186, 69-94, 1998.Coxeter,
H. S. M. 
." §14.2 in